Classifying PolynomialsPage
1
1
Slide 1
CLASSIFYING POLYNOMIALS
Slide 2
A _ is a sum or difference of terms. Polynomials have special names based on their _ and the number of _ they have.
POLYNOMIAL
DEGREE
TERMS
Slide 3
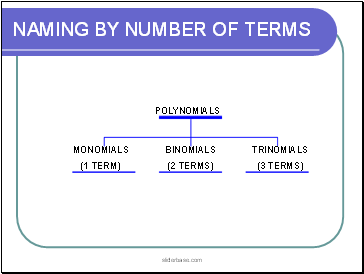
NAMING BY NUMBER OF TERMS
POLYNOMIALS
MONOMIALS
(1 TERM)
BINOMIALS
(2 TERMS)
TRINOMIALS
(3 TERMS)
Slide 4
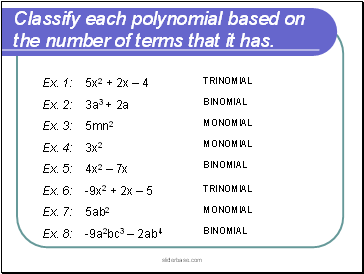
Classify each polynomial based on the number of terms that it has.
Ex. 1: 5x2 + 2x – 4
Ex. 2: 3a3 + 2a
Ex. 3: 5mn2
Ex. 4: 3x2
Ex. 5: 4x2 – 7x
Ex. 6: -9x2 + 2x – 5
Ex. 7: 5ab2
Ex. 8: -9a2bc3 – 2ab4
TRINOMIAL
BINOMIAL
MONOMIAL
MONOMIAL
BINOMIAL
TRINOMIAL
MONOMIAL
BINOMIAL
Slide 5
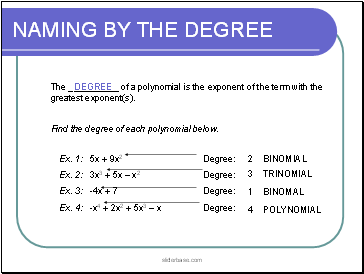
NAMING BY THE DEGREE
The of a polynomial is the exponent of the term with the greatest exponent(s).
DEGREE
Find the degree of each polynomial below.
Ex. 1: 5x + 9x2 Degree:
Ex. 2: 3x3 + 5x – x2 Degree:
Ex. 3: -4x + 7 Degree:
Ex. 4: -x4 + 2x2 + 5x3 – x Degree:
2
3
1
4
BINOMIAL
TRINOMIAL
BINOMAL
POLYNOMIAL
Slide 6
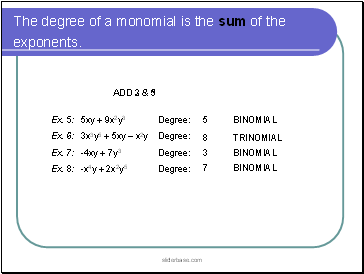
The degree of a monomial is the sum of the exponents.
Ex. 5: 5xy + 9x2y3 Degree:
Ex. 6: 3x3y5 + 5xy – x2y Degree:
Ex. 7: -4xy + 7y3 Degree:
Ex. 8: -x4y + 2x2y5 Degree:
5
ADD 2 & 3
ADD 3 & 5
8
3
ADD 2 & 5
7
BINOMIAL
TRINOMIAL
BINOMIAL
BINOMIAL
Slide 7
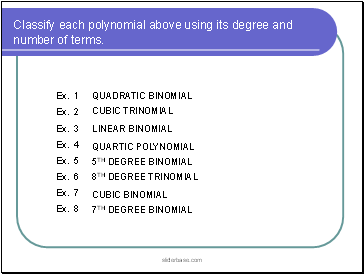
Classify each polynomial above using its degree and number of terms.
Ex. 1
Ex. 2
Ex. 3
Ex. 4
Ex. 5
Ex. 6
Ex. 7
Ex. 8
QUADRATIC BINOMIAL
CUBIC TRINOMIAL
LINEAR BINOMIAL
QUARTIC POLYNOMIAL
5TH DEGREE BINOMIAL
8TH DEGREE TRINOMIAL
CUBIC BINOMIAL
7TH DEGREE BINOMIAL
Contents
Last added presentations
- Newton’s law of universal gravitation
- Soil and Plant Nutrition
- The Effects of Radiation on Living Things
- Waves & Sound
- Newton’s Law of Gravity
- Static and Kinetic Friction
- Friction